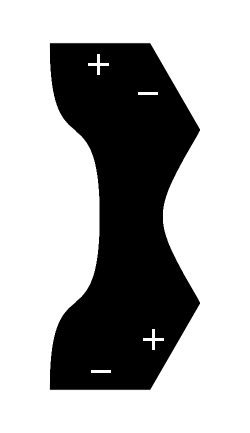
Print a bunch of copies of the shape on the right. 39 will do for a start. They need not be very large. Cut them out and arrange them such that as many "+" edges are connected to "-" edges as possible.
You should end up with a fractal structure.
This is an hexagonal aperiodic Wang tiling. I am unsure if i'm the first person to discover this particular type of tile. In any case, it's kinda neat.
If you put magnets on the flat edges matching the marked charges, put a bunch of the things in a box, and shake it, it will eventually self-assemble.
There is probably a three-dimensional analog of this shape. Molecules of that shape with appropriate charges would self-assemble into three-dimensional fractal structures. The resultant material would potentially be very strong yet of very low density.
Update, 4 July 2004: Andy Smith found a regular hexagonal pattern that can also be formed by this tile. The fractal pattern may still be the only infinitely extensible pattern if seeded with, say, a triangle. I'll have to look for some proof (or disproof) of this...